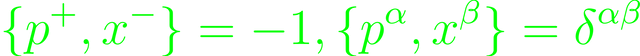String Theory

Classical Closed Bosonic Strings

Whew, that title is a mouthful, eh? So I visited a course on string theory and my professor wrote a book on the topic. I'll use this to accompany me through the semester to help my learning process, because how else am I going to hold myself accountable? Anyway, from what I understand, string theory is a bit of a unique beast in theoretical physics. Its not currently disprovable, and there is a good portion of the scientific community that is convinced that this is the only reason why string theory is still taught at university. Just as quantum field theory is using fields to represent everything, string theory uses strings, which has some frankly loopy-looking consequences, but the maths behind it are so odd and interesting that it makes it a wonderful experience to read about and study.

The simplest physical object to model without oversimplification is the relativistic particle. From the outset we begin with the classical approach to the equations of motion, that is the variational principle of least action, that is trying to map the motion along the geodesics on spacetime. However, we will run into some wacky dimension shenanigans in the future, so we'll stick to the most general case here, meaning instead of jumping to 4 dimensions, we will have to contend ourselves with having D dimensions in Minkowski space. That is the time (0-th) and D-1 spatial coordinates. We will have to make sure we keep the metric intact.

Variation of this action gives the equations of motion. The form isn't too important for us, so I won't include it here, but I will have to note that the premise here assumes a massive particle. Inserting a massless particle will make the action trivial and the subsequent suppositions pointless. The equation of motion resulting from the variational principle here is also non-linear and thus maybe a little intimidating. In fact, it's so intimidating that we will make significant efforts to not have to deal with that problem. We use an equivalent description for particles that may or may not have mass.

The second equation gives a formula for massive particles, the third is effectively identical to the equation of motion the first action gives us, which is good, since we wanted the two to be equivalent. I, for one, am still scared of that last one, so third formulation, here we come. Before that though, the second action is invariant to a reparametrization for some arbitrary real function.

The new parameters are straight up inserted into the second action to get the third, which yields the last line of equations of motions, the latter one being more of a constraint that we demand all our solutions satisfy to be valid within string theory.

We know from previous studies that solutions to the system will appear in phase-space, which has a momentum axis for every spatial axis. That means that in D-dimensional spacetime, we have a 2(D-1)-dimensional phase space. Similarly, the Minkowski space has 2D parameters, one being eliminated by the constraint and one can always set a starting condition that eliminates a second, leaving us with 2(D-1) phase space dimensions.

For the relativistic string, we will have to reevaluate the geometry of the motion, and then change the action to fit this. Before we do this though, we should talk about the geometry of the strings first. Intuitively we all understand how a string looks like, and technically, that's not wrong. We want to use an empty cylinder that we squash and stretch, only retaining the topology up to the edges. We only need to parametrize them for the cylinder height and angle to identify a spot on the manifold. We use this to define the Nambu-Goto action.

where a, b iterate over {0, 1}. Greek letters denote spacetime coordinates. This, too produces non-linear equations of motion, so we do what we did before, adapting the action to the dynamic metric fitted to the world-sheet. This yields the Polyakov action.

Upon eliminating world-sheet dependency, the Polyakov action would revert back to the Nambu-Goto action. The Polyakov action, too has symmetries similar to that of the third action we worked with, Since we already know the way by construction that it's invariant over parametrizations on the world sheet.

The only thing we need to do now is to take the cylinder-shape of the "field" into account. This would give a free field action with another constraint, which follows from the constraint equation we got from the previous paragraph.

This action fully characterizes the string and so it can be used to model it within a free field, hence the (FF) in the index. From here, we can attempt to quantize the string using the familiar tool of the anti-commutator. The classical equation of motion is relatively uncomplicated, just equating what's effectively the D-dimensional d'Alembertian to zero. Such objects solve the 2-dimensional wave equation and describes a superposition of two counter-propagating waves. Such waves don't influence one another at the generator, so splitting the system into left-propagating part and a right-propagating part is unproblematic. A few little arithmetics leads to a general solution on the cylinder

with the following definitions

This already looks very familiar in construction, as it gives us creation/annihilation operators, and imposing the standard Poisson structure where

and the rest vanishes, yields the canonical Poisson structure for the solution.

From here, one can identify the stress-energy-tensor by the usual rules of deriving it from the Lagrangian density. It should retain its usual properties, however from the construction of the two counter-propagating waves, the energy stress tensor can give expressions for the two modes, as well as their anti-commutator.

Free Bosonic Quantum Field Theory

Stepping away from strings for a bit, the quantum field theory of free bosonic fields is attained chiefly by the usual correspondence principle from the Poisson-Bracket to the commutator. This effectively removes one imaginary unit from all the elementary commutator relations of the previous chapter. We have to construct a new space to operate in for our theory, though. We keep the usual state-algebra along with creation/annihilation rules, so most things come out normal, until we try to evaluate

which is a non-positive-definit bilinear form, disqualifying our space of operation from being a Hilbert space. Before we continue, we perform a Wick rotation on the time coordinate and insert that into the quantum field X from the end of last chapter. A Wick rotation is done in the following,

but I won't do the entire calculation here, they are relatively straight forward.

From here we define the derivative fields J of X, and the vertex operators V.

The vertex operator applied to the ground state |0> evaluates to |k> through straight forward computation. With these fields we can derive correlation functions and make some statements about the Stress-Energy Tensor. Let's start with the correlation function first. This is mostly computation, so I'll sketch out the steps, and encourage the reader to do them for themselves. First, the products of Vertex operators are

Note the coefficient on the right side. The exponential form follows mainly from reordering of the products. It shows up in the commutator of the right-side and left-side propagators. It also shows up in the correlator that follows directly.

The form of this constant is of course very intuitive, considering this is supposed to be modelling the transition between world-sheets, so something scaling with their distance is sensible to have. The currents can also be decomposed into a creation and annihilation part (similar to the right-side and left-side propagators), each with its own set of non-trivial commutators.

The quantization introduces a form of the Stress-Energy Tensor that can be written as the normal ordered squared current (up to a factor 1/α'). Through the mode modes, it can however still be written as the Laurent expansion in the world-sheet coordinate z, which in turn introduces a Virasoro Algebra for the modes.

c is considered the central charge and is determined by the background. The second term is the central extension. If it vanishes, the algebra would define the Witt Lie-Algebra.

Covariant Quantization

This is where the Constraints we derived from the actions come in. We keep in mind that our free bosonic field theory has a cylindrical topology. We denote the constraints as l, which is mainly so we can use it for arithmetical considerations. First, constraints have non-trivial Poisson-Brackets, so those constraints should evaluate to zero, if we want to retain invariance of our problem. This isn't generally surprising though, as the independent constraints were always expected to be submanifolds in the solution space, i.e. in the phase space. Once the constraints are set to zero, the quantization can be applied. Alternatively, the quantization could be applied first, transforming the constraint function into linear operators. Through the condition that the constraint operators applied to the state, we can arrive at the same reduced Hilbert space. The first method is the "covariant quantization", the latter is the "light-cone quantization". We then can write the quasi-Hilbert space explicitly as

The N differentials given by the constraints cancels N of the degrees of freedom, so the resulting space is d-N dimensional. We want to implement the constraints explicitly into the problem we have defined so far. In the phase space, we operate with the canonical Poisson structure with the constraint

Quantizing a 2-dimensional system of this kind (so the minimal phase space) and the constraint operator, give

Extrapolating this into higher dimensions is relatively trivial. Alternatively, we can use that the constraint given by which happens to define the functions running on the light cone. We first consider the covariant quantization of the closed bosonic string. We take the same geometry. We construct an n-indexed set of constraint operators L and their complex conjugates, which will work as analogues to the constraint equations and their complex conjugates. This choice is somewhat complicated by the normal-ordering dictating the form of the zeroth constraint operator, which might differ from the quantum version of the constraint equation. It's identified up to some finite shift

This constructs a state space for the bosonic string.

We then apply the constraints. This is done for all n > 0, which allows us to get away with a weak form the constraint

Applying the restriction that these constraint operators with the index n ≠ 0 vanish. This is a weaker form of the vanishing of matrix elements. This introduces a space that still contains negative norm states, which is an issue, considering we wanted this to be a Hilbert space of wave functions. To filter out the negative norm states, we can look for solutions to the equation. Since k are the states, we will have to find some fitting D and a. It turns out that these are D=26 and a=1, which (unfortunately) implies that our space has 26 dimensions. Sketching out the proof can be done "by example", rather than by rigorous proof, through selecting a state and making sure that the polarizations of the eigenstates are space-like. As all constraint operators for n ≠ 0 applied to the state vanish, the polarization is perpendicular to the state, but and the n = 0 constraint returns the eigenvalue a. From this last constraint we gain an equation that, along with that a ≤ 1, we get a strong suggestion that a = 1.

By trying out D=26 and a=1, we can construct a space and find that after application of the constraint of the closed bosonic string, those states are divided out whose norm vanishes. The resulting space then finally has the positive definite bilinear form that we expect of a Hilbert space. The vibrational modes of closed strings can be modeled as an infinite set of particles. This, along with the wave functions of the bosonic strings can give a description of the mass spectrum. The simplest expression of mass is the negative square of the particle momentum. The Varisoro constraints have been constructed to commute with the momentum. The squared mass then is a well-defined operator. We use the generator representation of the 0 constraint operator.

where N is the number operator of its index. The same formula exists for the conplex conjugate. This gives us a negative ground state, which is an indicator for tachyonic excitation, which so far isn't great, but will be ignored until later time. Massless states are found for n ≥ 2. Further can be found out through the inspection of the lowest-level states without oscillation. For such states, the field is a superposition of eigenfunctions of the momentum operator.

The second of the equation gives a constraint for the amplitude inherited from the previous set of equations. The field amplitude is mostly zero, except for a squared mass of -4/\alpha'. Upon inspection, we can recover the Klein-Gordon equation for our field.

Through representation theory and application of an amplitude matrix on a state, we recover a solution for the light-like momenta in SO(1, 25) transformations. The momentum k takes the form k = (1, 1, 0, ...0). The solutions for these wave functions live in the GL(24), which suggests that the wave functions have actions in SO(24), which is a subgroup of SO(1, 25), while leaving k invariant. Representation theory can also be used to decompose SO(24) and identifying the resulting subgroups into a symmetric traceless part representing spin 2 particles (gravitons), an antisymmetric part mapping onto 2-form fields (B-fields) and the Identity, which maps to the dilaton.

We return to the tachyonic characteristics we've put off earlier, through inspecting the scattering amplitudes. For this, a few short words on the geometry of the string might be useful. Assume a closed string scattering amplitude with M external legs and without holes. We adopt much vocabulary from that of the Feynman diagrams to keep it simple. Such a string can be cut into M-2 elementary vertices, each weighted with an interaction factor. The M-point vertex then has a factor of the interaction constant to the power of M-2. The closed string amplitudes then have an interaction factor. We treat the diagram similarly to the Feynman diagrams, with the an appropriate field V, that lifts ground states into higher excitations and integrating over all insertion points. This still grants infinite solutions, but those can be whittled down by applying symmetries of the correlation function. This is a new addition to this object. By construction, the correlation function can't change as we translate over the insertion points by some complex constant b. With a, b, c, d complex parameters,

The amplitude then is proportional to the volume the entire space SL(2, C), so this volume needs to be respected when normalizing. The result will be finite.

Light-Cone Quantization

The light-cone quantization will further cement our choice of 26-dimensional strings with the normal ordering constant a = 1. We shall jump straight into it instead of recapturing the problem, as it's presumably not too far back that we've seen why we can choose this quantization over the covariant one. We begin with a 2-D dimensional phase space generated by the coordinates and associated momenta. The standard relativistic constraints remove 2 degrees of freedom for each particle. Each additional constraint generates shifts in the world line τ, which can be further explored through gauge theory. We define the following for later convenience

with ɑ ∈ {2, ... D-1}, which delivers further poisson-brackets.

From here, the observables of the system exist in the space of functions that commute with the constraints. To get there, we assume that

Important observables include the generators of the Poincare Algebra

We use these to generate infinitesimal Lorentz transformations and translations, so these are very much essential to describe a relativistic system. However, the Poincare symmetry was broken by light-cone gauge condition, so it makes sense to check the symmetry operators again. The retention of Poincare symmetry can be verified by rewriting the Lorentz operators through position and momentum operators and checking their commutation relation explicitly. It comes out fine. From here, the further quantization follows the standard procedure, associating the position operator with the momentum operator and transitioning to the quantum mechanical commutator.

To model relativistic strings, we need to find the appropriate constraints, preferably using the same tools that we used to express the constraints for the closed bosonic strings, these being the Laurent coefficients. We express the creation and annihilation operators

This establishes a Witt-Poisson algebra. The light-cone quantization of the bosonic string then is relatively low-maintenance from here on. The states can be constructed by the outer product of Hilbert spaces constructed from ground states. Those are generated by the transverse creation operators. The resulting state space and squared Mass operator is defined

We apply the constraint from the covariant quantization to relate the dimensions to the normal ordering constant.

We remind ourselves that we wanted the normal ordering constant to be 1, so the dimension has to come out as 26. Otherwise, the Poicare algebra would break again, and our state space wouldn't be valid for the lack of Lorentz operators. The computation is mostly contraction of sums and definitions, so I'll skip over it at this point.

Open Bosonic Strings

Modeling open bosonic strings will merit revising the geometry again. In the most generalized case, the problem will take place on some series of world-sheet manifolds, so it makes sense to map the components of the string onto such a space-time manifold. This of course will mean that the action needs to be adapted. Closed strings consist of an infinite strip 𝛾 = R × [0,π], with the string theory standard constraint. The boundary of the strip will want explicit solutions. We want to start off with the Polyakov action again, but because of the openness of the problem, we require some extra constraints. We find those by taking the variation of the Polyakov action and setting it zero at the borders of our strings. It's a straight-forward computation, if one keeps in mind that the variational operator can be pulled into the integrals and past the derivative, as long as it's with respect to different variables. This yields the Neumann boundary condition and the Dirichlet boundary condition respectively

each of which can be applied separately. It tosses out the extra dimensions that were added to the problem by the open boundaries.

The quantization with both conditions tends to follow the same motions as that of the closed strings, with some extra geometric requirements attached. Because of the boundary conditions being set to 0 at 𝜎 ∈ {0, π}, non-trivial solutions can only live in the positive half-plane. One can always check the validity of a solution by checking the results on the boundary, i.e. removing the imaginary component from the argument z.

The meat of the subject is looking at the mass spectrum of open bosonic strings.

As mass is tightly coupled with energy, the general approach should be to first identity the energy. For that, we use the same Virasoro generator that we used on the open string, extended with another term.

where Δ is the transverse distance between two branes. The new terms appear only in the diagonal of the higher orders. The openness of the string makes passing through branes a non-trivial matter. For a better look at the brane-string interaction, we want to check out the changes made to the state space. We can take the state space of the closed string and first impose the constraints. Open states need to be connected to at least one brane on either side for the oscillation expression to make sense, so we can safely remove all null states from the state space as well. The mass operator will commute with the constraints, because otherwise it wouldn't neatly apply to the string's wave function. It can be given explicitly by

The tachyonic character for small brane distances is still here. That state describes a scalar tachyon forming between two branes only if they are close enough. The ground state operator applied to a state will recover the Klein-Gordon equation by way of the mass shell condition. Mass then is an expression of string tension between branes, the anchoring function of which are the originator of said tension. Mass shifts are the energy required to push branes apart against the string tension.

Tree-level diagrams of open strings let us slowly transition into familiar territory of Feynman diagrams. Because of the way strings are constructed, i.e. the absence of defined vertices, where coupling constants would enter the product, that is a new mechanism that needs to be re-established, since we can't really avoid the coupling constant g anyways. We want all tree-level amplitudes to come with the same power of g. For this to make sense even under tree-level diagrams with multiple vertices, each leg must absorb one power of g, and open string diagrams start off with a power of -2 to begin with. A diagram for open strings at tree-level with M legs, then has a power of M-2 of the coupling constant g. The points where string runs through branes are the insertion points. They are important and substantial. Having them on the boundary makes them difficult to evaluate, so we just include them implicitly by integrating over them.

We adapt the necessity for boundary conditions on the integral and define the usual quantities.

This definition doesn't yet take scattering into account, so there are some small correction to be made here. Approaches to scattering chiefly takes into account the symmetries, which get handed down to the correlation function. For one, the correlation function is invariant under all rational transformations of the real line. Simple translations are included in those. They generally have 3 free parameters, so we place them in the three-parameter group. On a plane, three insertion points can be fixed at ∞, 0, and 1 respectively, then the rest remain to be integrated over. We'll keep this as convention moving forward. Tree-level amplitudes for open strings on a single Dp-brane then are writte as

We've dealt with a single Dp-brane, now we want to layer them on top of one another. For all intents and purposes, we treat them as being in the same position. Here, we will have to introduce an effective colour charge to mark which brane the string is attached to. We can use a matrix with only one non-vanishing entry to mark this colour charge. It extends the state space for coloured open strings by a tensor product with some N × N matrices. Colours can't change along boundaries, so the amplitude of the string should remain proportional to the trace of the colour matrices of external states. We call these matrices Chan-Paton Matrices. Taking their trace will suffice in setting the colour-choice. It takes the product of the Chan-Paton matrices for each index pair, and takes the trace of the result, as a factor to the amplitude.

On the gauge-theory side of things, strings on Dp-branes are originators of vector fields with p - 1 transverse degrees of freedom. We absorb the Chan-Paton field into the particle field. We keep with a standard gauge transformation, the non-Abelian guage theory. In essence we need to define a new covariant derivative operator. We require that the covariant derivative transform the same way as the field and we want the transformation matrix and the derivative to switch. That induces the particle field transformation and the field strength tensor transformation

We keep this in mind as we continue.

We start off with modes of the wave function. They have representations of the form

The boundary vertex operators remain the same as we've known. A 3-point vertex then only needs to multiply all of those together. It comes out as follows

So far so good, that doesn't tell us anything new so far. We keep with the familiar commutators for the right and left moving wave operators and the vertex operators. These can be inserted into the propagator term with very little effort. We again fix the first (and only) three points to ∞, 0, and 1 respectively, hence removing all the u-dependencies. We turn out with a lot of set, but uninteresting functions in the propagator, which includes cyclic permutations of ξ, so we write it as C, which is antisymmetric with respect to the latter two indices. What would have vanished before colour-charge considerations now turns out a direct contribution term from the Chan-Paton traces.

Free Fermionic QFT

This is still part of string theory, I promise. We've so far only looked at bosons, and on paper fermionic strings will work very similarly, though we have to construct them a little differently from the outset. This construction is based in quantum field theory, where fermions tend to feel at home the most (at least it looks like it to me). For this purpose, we take the action of a fermionic field

and map it onto the geometry we've used so far. We keep in mind the spinor representation of the field and we want the dirac matrices ρ to satisfy the dirac algebra. To get those sweet boundary conditions that string theory lives off of, the action needs to be variated, and of course set to zero. This yields the following

which will act as the equations of motion for the fields. This means that the fields are only dependent on a single light-cone coordinate. This is eerily similar to the behavior of the Virasoro algebra for the bosonic strings. Because of 2π-periodicity, there is either the option for the field to be symmetrically periodic (Ramond sector) or anti-symmetrically (Neveu-Schwarz sector), each can be applied separately to either the left or right movers, yielding either RR, RN, NR, NN boundary choices. We take the normal definitions for Π and the energy-stress tensor from QFT, but note that the latter also has a representation through the Fourier modes l, which we have already seen before.

The two sectors have different field representations with respect tot their Fourier modes, while obeying the anti-commutator relations we're used to from the bosonic string.

In terms of the space, each sector comes with its own unique ground state. In the N-sector, we define for this purpose more operators derived from the fermionic modes. These follow directly from the Dirac algebra relations.

where b are the fermionic modes. They are, as always, subject to the typical algebra. The space these span contain D/2 fermionic creation operators, and as always, that becomes the exponent to the base of 2 for the dimension of the state space. Defining each of the state spaces with the four sector-combinations, we obtain a full space with 4 discrete sectors. These describe the state space of the closed fermionic field (CFF). The Virasoro generators change under this treatment, because not all of the annihilation operators commute anymore. Their commutator shows up as a sum. For the Neveu-Schwarz sector, the mode numbers come in halves.

For a full theory, we first want to bring both the bosonic and fermionic fields into one action. That's as easy as adding them together. However, since they want to interact, we have to introduce supersymmetry transformations

The newly introduced ϵ is a Grassmann constant.

We can especially say that the stress energy tensor can be constructed from a sum of both the fermionic and bosonic one. The superpartner (we'll get to that another time) is

For a full state space, we construct it from the state space as we know it and take the cartesian product with that of either sector combination. Notably, the charge adds up over the product and we call the resulting product N=1 super-Virasoro algebra

where each mode of G is constructed through the modes a and b

D will turn out to be 10, which we'll accept for now, the proof will presumably show up later down the line.

Supersymmetry in 10 Dimensions

The term "string theory" is really not the entire story. If that were all there was, we'd be more or less done, but in the previous chapter we've seen that to build a model that includes both bosonic and fermionic particle strings requires we consider supersymmetry into the model. It suggest that something is missing in the model. And then there's the fact that in the N = 1 superalgebra, there was a different dimension attached than we were used to. 10 dimensional is a little odd, no? There's also a number of particle states that are missing, which can be explained using supersymmetry.

We take the 10 dimensions as correct and continue in the corresponding Minkowski space-time. Through representation theory, that space can be decomposed into the Poincare algebra SO(1, 9) ⋉ R(1, 9) with the Poincare generators as is expected from Minkowski space-time. Refer to previous chapters for their commutator relations. I was not a diligent student during my bachelor's so the construction of both these spaces wasn't clear to me, and as such I'll indulge myself and go into detail what SO(1, 9) is. SO(n) is the space of orthogonal matrices with determinant 1. SO(n, m) still has determinant 1, and is still a subgroup of O(n, m), which is the orthogonal group of non-degenerate quadratic forms. Okay, what is that? We established that we wanted to move in a 10-dimensional space. Let's generalize and call the dimension d again. We require that n + m = d, and the matrices in SO(n, m). A quadratic form of that type is supposed to be written as a sum of both n and m squares. In short: It's a 10-dimensional matrix that is also a sum of squares.

Our construction has little to do with that though, so let's take a look at what's in it, or rather how we construct it. We borrow heavily from algebra here, so it might be good to have the basics for that down. First will be the semi-direct product. It maps a group action from SO(1,9) to R(1,9), and with that come orbits. The ones we need are going to be the trivial orbit and the orbit that retains the square momentum be equal to the square mass of the particle. Crucially then, each mass has its own orbit. The former is just there as a formality that'll aid us in completing the classes of representations, the latter contains the momentum of multiplets with mass m. For the purposes of avoiding the generalization (which is overkill at this point), we choose a point q in any orbit. The representation won't depend on this choice, it'll just be convenient to have around. Note the stabilizer of that point is the subalgebra of all Lorentz boosts and rotations, leaving it invariant. Write it as g, which then induces a subalgebra g ⋉ R(1, 9) on some vectorspace V.

Following up from the QFT segment, there is of course a way to utilize the spinor matrices to build a representation of the Poincare algebra. The basic idea for this is to go through the Lorentz algebra first. Using the γ matrices familiar from QFT, pairs of dimensions can be used to form one creation and one annihilation operator, so in 10 dimensions, that gives 5 creation operators. Note, that this will give us 11 γ matrices in total (0 - 9, 11). The Lorentz generator commutes with them, and the γ matrices retain all their usual properties. That decomposes the representation space into two inequivalent representations of SO(1, 9) through the projection operators Π

This splits a D dimensional representation into two D/2 dimensional Weyl representations. This method should look familiar from QFT. It's true that this is only really applicable for even-dimensional representations, but in praxis, we only ever really work with those anyways. If we move to extrapolate the D = 4 case into a general approach, then it's helpful to construct a matrix B that is the product of all odd-numbered gamma matrices in ascending order of index safe for one. B will have the following properties.

The generators of this resulting space are (iΣ Ψ)* = B(iΣ Ψ). This gives rise to the further decomposition into Majorana-Weyl multiplets, which only exist in 2 + 4n dimensions. Then, dimension 10 makes a lot of sense. It might of course also be a number of other dimensions, but from supersymmetry, dimensions above 11 will automatically decomposes into smaller spaces, dimension 2 is too small to make two decompositions that make sense, and dimension 6 still gives half dimensions for the second one. If we take D = 10 as a given again and trace this down, we will note that both decomposed S are of dimension 16, and they decompose into two 8-dimensional subspaces through the group actions.

Returning to the 10-dimensional superalgebra, we remember that what we defined was the N = 1 Poincare superalgebra. Much of the preceding paragraphs functions as an extrapolation from the construction of the N = 1 Poincare superalgebra, so to expand on the construction, we're only really interested in the anti-commutator for the supercharges. It's not quite the same thing, but the inclusion of which has very similar reasons to the anti-commutator construction for the gamma-matrices in quantum field theory.

with the charge conjugation matrix C. So far so good. We did however establish that there is something called the N = 2 Poincare superalgebra. Now what is that? Instead of one Majorana-Weyl supercharges, we have two. In the supercharges, a reaches up to 16, as was expected, and we introduce another index that goes from 1 to 2 demarcating the two different supercharges that will be included in the system. Decompositions in 16-dimensional multiplets are intuitive. We differentiate between two types of Poincare superalgebras. For this, we keep in mind that in the N=1 superalgebra, the multiplet has 2 different representations, namely 16 and 16'. Mixing them gives what is referred to as a type IIA superalgebra and having either 16 ⊕ 16 or 16' ⊕ 16' gives a type IIB superalgebra. type IIA superalgebras have transform in two inequivalent Majorana-Weyl representations of SO(1, 9). They hold the commutation relation

Type IIB reduces this to an easier relation.

Each of these relations give their own 10-dimensional superalgebra, meaning the N = 2 Poincare superalgebra induces 3 different 10-dimensional superalgebras.

Within the N = 2 superalgebra, the massless multiplets will of course experience changes once again, as they are derived from the commutator relations, of which we have several now. By definition, supercharges act trivially on momenta, so all but the 0th index of the momentum will be contributing to the commutator relation. That is the constant value on V. It reduces the space to that, on which the square momentum is null, which still exists on SO(8), but decomposes slightly differently. These representations are connected mainly by the supercharge actions.

In sum, there are 32 supercharges, which obey the commutator relations from the previous paragraphs. As in the N = 1 superalgebra, the groups of 16 supercharges can be decomposed into two sets of 8 creation & annihilation operators. The vector space can then be decomposed into groups of the size of states constructable from the ground state with 0, 1, 2, ... fermionic creation operators. 0 gives the dilaton, 1 the dilatino (which is symmetric in this property, so does the 7), the 2 gives the KR-field, 3 the gravitino (which is also symmetric, so does the 5), the 6 gives the 2-form and the 8 the 0-form. In between is the 4-form, which is the graviton, but has the dimension of 70, which needs to be constructed from 4 creation operators and is the only group not irreducible. All in all, the supermultiplet contains 128 bosonic components for the dilaton and four fermionic multiplets (for the symmetrically constructed groups). For the type IIA superalgebra the analysis is very similar.

Physics 2023, 34: Construction of Type IIA/B Superstrings

The construction of superstrings follows conceptually from the imposition of super-Virasoro constraints, before requiring further conditions from the states. It's not essentially very different from the construction of bosonic and fermionic strings starting from the free field theory. Instead of the previous constructions, i.e. singlet/multiplet in free-field theory, now the space for quantization emerge from the supermultiplet in the Virasoro field. We've characterized these through the fields and their superpartners, which act as constraints upon the field equations. A lot of these computations are analogous to the earlier constructions. Right-movers of the R-sector was previously constructed as

where the left side carries the N=1 superconformal algebra action with the familiar generators. To avoid the tachyonic states, the weak constraints of the bosonic string will be stripped of the normal ordering ambiguity in the ground state generator. Because of the ground state superpartners being constructed without normal ordering, and the axiomatic constraint that the ground state superpartner annihilate all physical states, the current G arises from the quantization with the commutation relation

which gives a quantum version of the classical constraint of the mode operator. We want to impose

for all physical states. This leaves the dimension parameter to be set. Like before, we discount dimensions with negative norm states, which ends up being D = 10. To verify that this dimension is good, we check the wave functions after removal of zero norm states.

By this, the squared mass spectrum is bounded by below by 0 and the R-sector has no tachyonic modes. We adapt the five superstring creation operators from the supersymmetry chapter and apply the constraint.

This construction retains the proportionality to the annihilation operator, so the state space still decomposes into 16 states, and the subsequent representations of even and odd spinor representations. The even ones correspond to the dilatino, and the odd ones are a complementary representation that helps construct the non-chiral fermionic component.

The Neveu-Schwarz sector's state space is constructed very similar to that of the Ramond sector's. However, because of the half-integer indices in the superpartner modes, the mass spectrum turns out with a tachyonic component again.

The massless states on the other hand are relatively uncomplicated and lands the state as an element of a 8-dimensional vector multiplet. Apart from the tachyonic mode, the representation of the massless state as an 8-dimensional vector field makes direct space-time symmetry between massless bosons and fermions impossible. Note that

The tachyonic mode ends up so that it doesn't contain fermionic creation operators, and the massless vector multiplet in the N-sector only has one fermionic mode. Further, in R-sectors one of the multiplets contains an odd number of fermionic creators and the other has an even number. Taking out all but the states that have odd numbers of fermionic generators would remove the tachyonic mode and one fermionic octoplet, which also surprisingly remains functional through the consistency checks. For this, the the states with an even number of fermionic creation operators have to be projected, using the Gliozzi-Scherk-Olive projection.

We keep in mind that the full state space is constructed of an outer sum of each state space with all permutations of the right/left moving N and R-sectors. This results in one of two constructions for the projection operator

The index indicates the type II-Superstring that survives after the application of the operator. The type IIB GSO projection on the NN-sector has following (de-)composition

1 gives the dilaton, 28 the Kalb-Ramond field, 35 the graviton. In RR

1 gives the 0-form, 28 the 2-form, 35 the self-dual 4-form, all of which construct fields. These sectors represent space-time bosons, and the as of yet undiscussed sector combinations the space-time fermions. RN-sector

where the 8 gives the dilatino and 56 the gravitino. NR-sector

has the same elements, but because these are spaces we're working with, the order on the left matters a lot.

The type IIA projection functions equivalently, and the NN-sector is exactly the same as it is for the type IIB projection. There are changes in the RR-sector

with 8 giving the 1-form RR-field and 56 the 3-form RR-field. RN-sector gives

with 8 giving the dilatino and 56 the gravitino. Both of these are left-handed for those whom that means anything to (I'm unfortunately not one of them). The NR-sector constructs

where 8 is again the dilatino and 56 the gravitino, both of them right-handed. This defines the type IIA theory as non-chiral and N=2 supersymmetric. This approach is paralleled by a the Green-Schwarz formalism, which will probably remain undiscussed in this exercise. The parallelity of the approaches is not dissimilar to the parallelity between the quantizations at the very beginning. Skipping a bunch of computations, that follow the same general steps as the ones done to determine the gauge theory for the strings before supersymmetries. That will lead to a actions for both exclusively N- and exclusively R-sectors. For the exclusively N-sector, these are identical across both type II supersymmetries. These will pave the way for a supergravity theory.

The exclusively R-sector in type IIA gives the action

Even-numbered indices of F denote the field strength of the RR potentials in type IIA theory. The final term (Chern-Simons) describes the gauge field contribution to the action. Making sense of the expression requires cheating a lot of the wedge products using Stoke's theorem, Leibniz rule and Bianchi-identities, and even then they don't turn out terribly great readable. In the type IIB theory, the RR potential becomes

It functions the same way in construction as the type IIA action.